Generalized Mandelbrot Sets and Moduli Spaces
of f(z) = z n + c
Pure Mathematics Department, University of Waterloo, Ontario, Canada
Mathematical Description
Polynomials f(z) = zn + c
The polynomials f(z) = zn + c (for n>1) are those with precisely two critical points on the Riemann sphere, at 0 and infinity.
Generalized Mandelbrot Set
The generalized Mandelbrot set of f(z) = zn + c consists of those complex values c for which the Julia set of f(z) is connected.
Moduli Space
A Mobius transformation that fixes 0 and infinity is of the form z -> wz.
Two polynomials zn + b and zn + c are conjugate by such a transformation if and only if b = wc, where w is an (n-1)st root of unity.
Hence the polynomials are conjugate by a Mobius transformation, and so have the same dynamics, if and only if b(n-1) = c(n-1). If we identify all the parameters which give these conjugate transformations, we obtain the moduli space, which can be identified with C = c(n-1).
The two polynomials zn + b and zn + c are conjugate by complex conjugation if b and c are complex conjugates.
It follows that the dihedral group of order (2n-2), generated by a primitive (n-1)st root of unity and complex conjugation, acts on the generalized Mandelbrot set. It can be seen from the pictures below that the symmetry group of the generalized Mandelbrot set is the dihedral group of order (2n-2).
References
- Alexander, C.; Giblin, I.; Newton, D. Symmetry groups of fractals. Math. Intelligencer 14 (1992), no. 2, 32--38.
- Milnor, John. Lecture on "Rational functions with two critical points".
Pictures
For various values of n, the following pictures show the Mandelbrot sets of f(z) = zn + c in the c-plane on the left, and the moduli spaces in the C-plane on the right, where C = c(n-1).
 |
 |
| Mandelbrot set for n = 2 | Moduli space for n = 2 |
 |
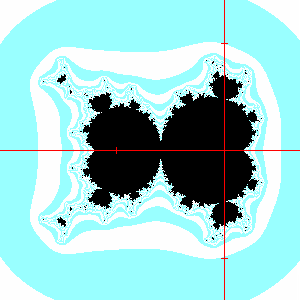 |
| Mandelbrot set for n = 3 | Moduli space for n = 3 |
 |
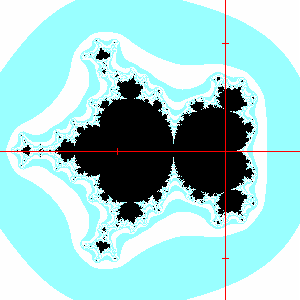 |
| Mandelbrot set for n = 4 | Moduli space for n = 4 |
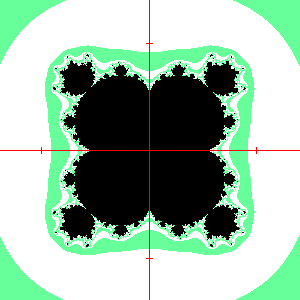 |
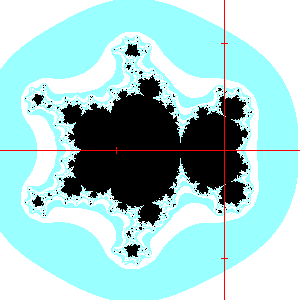 |
| Mandelbrot set for n = 5 | Moduli space for n = 5 |
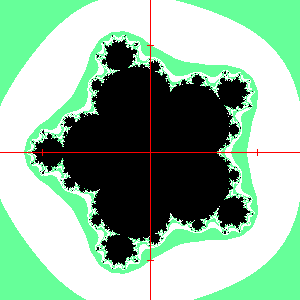 |
 |
| Mandelbrot set for n = 6 | Moduli space for n = 6 |
 |
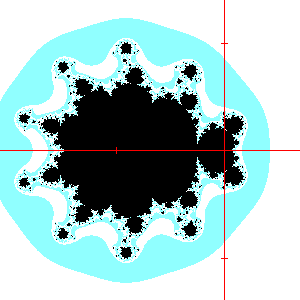 |
| Mandelbrot set for n = 9 | Moduli space for n = 9 |
 |
 |
| Mandelbrot set for n = 14 | Moduli space for n = 14 |
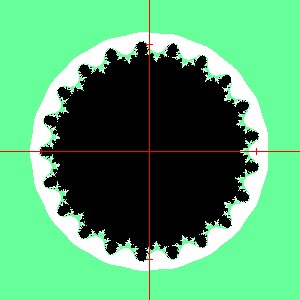 |
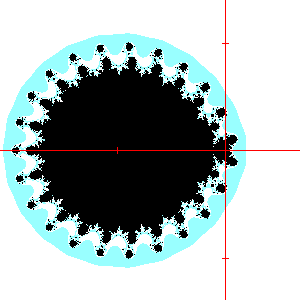 |
| Mandelbrot set for n = 22 | Moduli space for n = 22 |
© 1997 by William Gilbert
Back to the entrance of the Fractal Gallery of William Gilbert.

















